Fractals activity 2: Sierpinski’s triangle

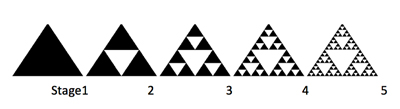
Sierpinski’s triangle

Background

Fractals are interesting objects involving many sequences and series. We are focussing on the Sierpinski triangle and in particular the perimeter and the area of it.

Perimeter

Above are the first five stages of the Sierpinski triangle. We start at stage 0, with an equilateral triangle with side length 1. In the first stage, each side has been halved, four triangles are created and the centre one removed. The outside of this removed triangle still remains and is part of the perimeter of the Sierpinski triangle. There are now three new sides, each of length 1/2. Each iteration removes a new collection of triangles.

Area of the triangle

At stage 0 we have an equilateral triangle with a certain area, say A. We can then find out the removed area at each stage in terms of A.

(Teacher note: To make this task easier, a value can be assigned to the initial area.)

Task

Fill in the table and answer the questions below about different features of the fractal.

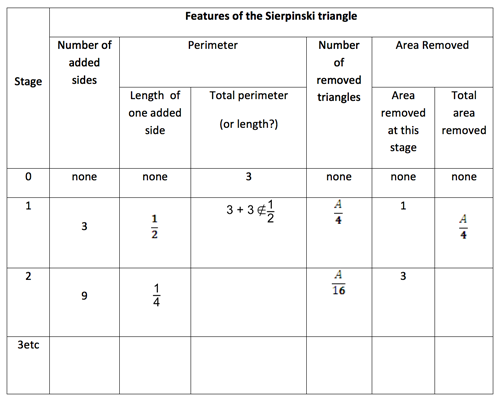
Table_Features of the Sierpinski triangle

Discussion

What patterns do you notice for the number of added sides, length of each added side, and total perimeter?
(Note: This involves two features; number of added sides and perimeter. Further, perimeter is a feature where summing the terms makes sense for the context.)
What patterns do you notice for the number of shaded triangles, area removed at this stage, and the total area removed?
(Note: This question involves two features; number of shaded triangles and area. Further, adding the sequences for area is a feature where summing the terms makes sense for the context.)
Investigate three different sequences or series over at least two features of the fractal. (You must include at least one sequence and one series.)
Describe how these features can be modelled using arithmetic or geometric sequences and series.
Find at least the first four terms and general term for the nth stage of your chosen features. This should include at least one partial sum.
Describe what will happen to the sequences and series as the number of iterations increase. (You may wish to use a sum to infinity where appropriate.)
Find the number of iterations or the stage at which you can guarantee the perimeter of the Sierpinski triangle is more than 100? (This is so you can demonstrate “finding the number of terms in a sequence”; 60 or 82.5 etc could also have been selected.)
Find the number of iterations or the stage at which you can guarantee the total area removed of the Sierpinski triangle is more than 0.7A. If you chose a feature other than the total length or area, then choose a limit that your chosen feature could reach. For this limit, find the number of iterations or the stage at which your feature first reaches this limit.